HEC-RAS is one of the most popular hydraulic models (numerical models). HEC-RAS has undergone important changes and improvements since HEC-RAS was first released in1995. In 2015 HEC-RAS 5.0 (with 2D capabilities) was released; this was a long-waited release. In a previous post we compared the 2D capabilities of HEC-RAS with Mike21. One of its main limitations was that it was limited to Newtonian fluids. I explicitly used the word “was” because at the end of 2020 it released its latest version (v 6.0 Beta), and this new version includes the capability to simulate Non-Newtonian fluids. In this post we evaluate the Non-Newtonian fluids capabilities by simulating the Brumadinho dam failure disaster.
What is the difference between Newtonian and Non-Newtonian fluids?
A
Newtonian fluid assumes that water begins to “deform” (strain) under any stress
(zero intercept on the strain-stress relationship), then the strain increases
linearly with the stress; and the ration strain-stress is the water viscosity.
A Non-Newtonian fluid is fluid that does not follow those assumptions. For instance:
- The fluid will resist an initial stress, and begin to deform only when the stress reaches a given threshold
- The strain-stress relationship is not a linear one.
There
different kinds of Non-Newtonian fluids depending on the sediment concentration,
such as hyper-concentrated flow, mud-flow, debris flow or clastic flow (to be
explained in a future post).
Previous studies simulating the Brumadinho dam break
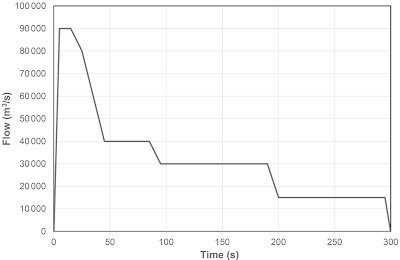
Lumbrosoet al., (2021) simulated the Brumadinho dam failure using EMBRE-MUD and Mike21,
and considering a domain based on a 5 m DEM. EMBRE-MUD provided an outflow
breach hydrograph with a peak flow about 90 000 cms.
The
mud-flow was simulated considering the Bingham model.
The calibration provided the following parameters:
- Fluid density: 1800 kg m3.
- Yield stress: between 750 – 1000 N m-2.
- Bingham viscosity: about 100 kg m-1 s-1.
- Roughness values between 0.063 (pasture), 0.031 (earth and mine site) and 0.167 (forest).
Raman and Liu (2019) performed a 2D flood simulation using HEC-RAS v5 (Newtonian approach). This study assumed a very high roughness (a non-real roughness) to retard the flood wave to replicate the flood arrival time. This study considered a domain based on the SRTM DEM.
Simulating Brumadinho dam failure with the new HEC-RAS v6.0 Beta
In
a previous post we demonstrated the capabilities of HEC-RAS to simulate 2D dambreak scenarios. In the present post we focus on evaluate the Non-Newtonian
capabilities of HEC-RAS by simulating the Brumadinho dam break, by and
comparing with results from other simulations.
The present simulation assumed the values calibrated by Lumbroso. The main difference is that Lumbroso considered a 5 m DEM, while we considered a 30 m DEM (SRTM).
Three
simulation were performed considering different approaches:
- Non-Newtonian fluid and Bingham. This simulation considered the values calibrated by Lumbroso et al.
- Newtonian fluid and n=0.03. This simulation considered a normal average roughness to show “what if” we assume a normal Newtonian fluid.
- Newtonian fluid and n=0.3. This study considered a very high roughness to replicate the arrival time. The simulation considered the roughness 0.3 proposed by Raman and Liu (2019).
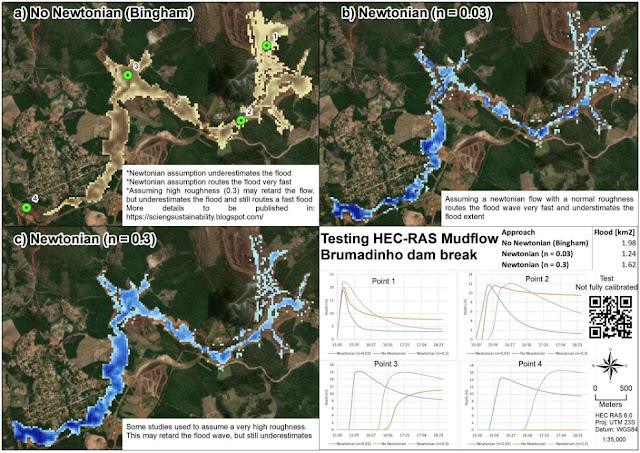
Results of the Brumadinho dam break 2D simulation
Figure
summarizes the result for the different simulation, and the youtube video shows an animated comparisson. It is clear that the normal
Newtonian approach underestimates the flood extent about 40%.
Even
tough the non-real roughness 0.3 improves the flood extent in the downstream and
the arrival time, it still underestimates the flood extent about 20%. This is
because the high roughness only retards the flood wave, but the flow is still
acting as Newtonian. This is better explained by the hydrographs. The
hydrographs of point 1 and point 2 show that the once the flood arrives, it
continues flowing; thus, the flood wave passes quite fast. On the other hand,
the Non-Newtonian approach shows that when the flood arrives it tends to stay;
it requires more stress to move downstream.
The
video animation also shows another important characteristic of Non-Newtonian fluid.
In areas when the terrain has a steep slope, such as point 1 and point 2, the
mudflow may move faster than the Newtonian fluid because the weight of the flow
is an additional force accelerating in the direction of the flow. On the other
hand, in flat areas the Non-Newtonian fluid moves much slower than the
Newtonian fluids.